Earliest Uses of Symbols for Constants
Last revision: June 23, 2017
π for 3.14159... Early writers
indicated this constant as a ratio of two values. William Oughtred
(1574-1660) designated the ratio by the fraction π over δ
in Clavis mathematicae. The symbolism
appears in the editions of this book of 1647, 1648, 1652, 1667, 1693,
and 1694 (Cajori vol. 2, page 9).
Cajori writes that "perhaps the earliest use of a single letter to
represent the ratio of the length of a circle to its diameter" occurs
in 1689 in Mathesis enucleata by J. Christoph Sturm, who used
e for 3.14159....
si diameter alicuius circuli ponatur a,
circumferentiam appellari posse ea (quaecumque enim inter eas
fuerit ratio, illius nomen potest designari littera e).
Cajori cites a note by A. Krazer in Euleri opera omnia as a
reference for the above.
The first person to use π to represent
the ratio of the circumference to the diameter (3.14159...) was
William Jones (1675-1749) in 1706 in Synopsis palmariorum
mathesios. It is believed he used the Greek letter pi because it
is the first letter in perimetron (= perimeter). From Cajori
(vol. 2, page 9):
The modern notation for 3.14159 .... was introduced in
1706. It was in that year that William Jones made himself noted,
without being aware that he was doing anything noteworthy, through his
designation of the ratio of the length of the circle to its diameter
by the letter π. He took this step
without ostentation. No lengthy introduction prepares the reader for
the bringing upon the stage of mathematical history this distinguished
visitor from the field of Greek letters. It simply came, unheralded,
in the following prosaic statement (p. 263):
"There are various other ways of finding the Lengths or
Areas of particular Curve Lines, or Planes, which
may very much facilitate the Practice; as for instance, in the
Circle, the Diameter is to the Circumference as 1 to 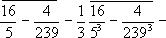 , &c. = 3.14159, &c. = π. This series (among others for the
same purpose, and drawn from the same Principle) I received from the
Excellent Analyst, and my much esteem'd Friend Mr. John Machin;
and by means thereof, Van Ceulen's Number, or that in Art.
64.38 may be Examin'd with all desirable Ease and Dispatch."
, &c. = 3.14159, &c. = π. This series (among others for the
same purpose, and drawn from the same Principle) I received from the
Excellent Analyst, and my much esteem'd Friend Mr. John Machin;
and by means thereof, Van Ceulen's Number, or that in Art.
64.38 may be Examin'd with all desirable Ease and Dispatch."
In 1734 Leonhard Euler (1707-1783) employed p instead of π
in "De summis serierum reciprocarum."
In a letter of April 16, 1738, from Stirling to Euler, as well
as in Euler's reply, the letter p is used.
In 1736 in Mechanica sive motus scientia analytice exposita,
Euler used 1 : π and "thus either
consciously adopted the notation of Jones or independently fell upon
it" (Cajori vol. 2, page 10). Euler wrote, "Si enim est m =
1/2 terminus respondens inuenitur π/2
denotante 1 : π rationem diametri ad
peripheriam." But the letter is not restricted to this use in his
Mechanica, and the definition of π is repeated when it is taken for 3.14159...
In 1737 Euler used π for 3.14159...
in a letter, and again in various letters in 1737, 1738, and 1739.
Johann Bernoulli used c in 1739, in his correspondence
with Euler, but in a letter of 1740 he began to use π.
In 1741 π was used in H. Sherwin's
Mathematical Tables.
Nikolaus Bernoulli employed π
in his letters to Euler of 1742.
Euler popularized the use of π by employing
it in 1748 in Introductio in Analysin Infinitorum:
Satis liquet Peripheriam hujus Circuli in numeris
rationalibus exacte exprimi non posse, per approximationes autem
inventa est .. esse = 3,14159 [etc., to 128 places], pro quo numero,
brevitatis ergo, scribam π, ita ut sit π = Semicircumferentiae Circuli, cujus Radius =
1, seu π erit longitudo Arcus 180
graduum.
The base of natural logarithms. This constant, 2.71828..., was
referred to in Edward Wright's English translation of Napier's work
on logarithms, published in 1618.
The first symbol used for the constant mentioned by Cajori is the
letter b used by Leibniz in letters to Huygens in 1690 and
1691.
Leonhard Euler (1707-1783) introduced e for this constant in a
manuscript, Meditatio in Experimenta explosione tormentorum nuper
instituta (Meditation on experiments made recently on the firing
of cannon), written at the end of 1727 or the beginning of 1728 (when
Euler was just 21 years old). The manuscript was first printed in
1862 in Euler's Opera postuma mathematica et physica,
Petropoli, edited by P. H. Fuss and N. Fuss (vol ii, pp. 800-804).
The manuscript describes seven experiments performed between August 21
and September 2, 1727:
For the number whose logarithm is unity, let e be
written, which is 2,7182817... [sic] whose logarithm according to
Vlacq is 0,4342944... [translated from Latin by Florian
Cajori].
Euler next used e in a letter addressed to Goldbach on November
25, 1731, writing that e "denotes that number whose hyperbolic
logarithm is = 1."
The earliest appearance of e in a published work was in
Euler's Mechanica (1736), in which he laid the foundations of
analytical mechanics (Maor, p. 156).
Maor writes (page 156):
Why did he choose the letter e? There is no
general consensus. According to one view, Euler chose it because it
is the first letter of the word exponential. More likely, the
choice came to him naturally as the first "unused" letter of the
alphabet, since the letters a, b, c, and d frequently
appear elsewhere in mathematics. It seems unlikely that Euler chose
the letter because it is the initial of his own name, as occasionally
been suggested: he was an extremely modest man and often delayed
publication of his own work so that a colleague or student of his
would get due credit. In any event, his choice of the symbol
e, like so many other symbols of his, became universally
accepted.
Ball says: "It is probable that the choice of e for a
particular base was determined by its being the vowel consecutive to
a."
According to Boyer (page 494), this notation was "suggested perhaps
by the first letter of the word 'exponential.'"
In a post in sci.math in 1995, Wei-hwa Huang wrote: "I believe
that e was not named because it was the first letter in Euler's
name, but rather because he was using vowels for constants in a proof
of his and e happened to be the second one."
In a post to a history of mathematics list in 1999, Olivier Gerard
wrote: "The hypothesis made by my friend Etienne Delacroix de La
Valette was that e was for 'ein' (one in German) or 'Einheit'
(unity), which would be matching the sentence Euler uses to define it
(whose logarithm is unity). As always, many explanations may be true
at the same time."
Several textbooks claim that the letter e was chosen to honor
Euler. Cajori has no information to support this claim, and in fact
the earliest uses of e were by Euler himself.
The early uses of symbols for 2.718... mentioned by Cajori are as
follows:
| 1690 | b | Leibniz | Letter to Huygens
|
| 1691 | b | Leibniz | Letter to Huygens
|
| 1703 | a | A reviewer | Acta eruditorum
|
| 1727/8 | e | Euler | Meditatio in Experimenta explosione tormentorum nuper instituta
|
| 1736 | e | Euler | Mechanica sive motus scientia analytice exposita
|
| 1747 | c | D'Alembert | Histoire de l'Académie
|
| 1747 | e | Euler | various articles
|
| 1751 | e | Euler | various articles
|
| 1760 | e | Daniel Bernoulli | Histoire de l'Académie r. d. sciences
|
| 1763 | e | J. A. Segner | Cursus mathematici
|
| 1764 | c | D'Alembert | Histoire de l'Académie
|
| 1764 | e | J. H. Lambert | Histoire de l'Académie r. d. sciences et d. belles lettres
|
| 1771 | e | Condorcet | Histoire de l'Académie
|
| 1774 | e | Abbé Sauri | Cours de mathématiques
|
| 1775 | e | J. A. Fas | Inleiding tot de Kennisse en het gebruyk der Oneindig Kleinen
|
| 1782 | e | P. Frisi | Operum tomus primus
|
| 1787 | c | Daniel Melandri | Nova Acta Helvetica physico-mathematica
|
The term Napier's constant has been suggested for 2.718...
The name Euler's constant may be inappropriate for this
number, as the number was known before Euler's birth and Euler's
constant more frequently is used to refer to 0.577....
Benjamin Peirce suggested the innovative notation for π and e shown below:
 From J. D. Runkle’s Mathematical Monthly, vol. I, No. 5, Feb. 1859
From J. D. Runkle’s Mathematical Monthly, vol. I, No. 5, Feb. 1859
A somewhat modified notation, shown below, appears in the Century Dictionary (1889-1897) in the
entry notation:

Euler-Mascheroni constant. Leonhard Euler (1707-1783) used
C in De progressionibus harmonicis observationes,
Commentarii academiae scientiarum petropolitanae 7 (1734-35),
published in 1740, pp.150-161. Reprinted in Opera omnia (1)
14, pp. 87-100.
According to Cajori (vol. 2, page 32), Mascheroni used A in
Adnotationes ad calculum integralem Euleri (1790-1792).
According to William Dunham in Euler, the Master of Us
All (1999), Mascheroni introduced the symbol γ for the Euler-Mascheroni
constant. Dunham's source is "On the History of Euler's Constant,"
by J. W. L. Glaisher, which appeared in 1872
in The Messenger of Mathematics. In the paper, Glaisher does
not specify where Mascheroni used the symbol, but seems to imply it
is in Adnotationes ad Euleri Calculum Integralem, which
Glaisher indicates in a footnote is a work he has not seen but which
is referred to in volume 3 of Lacroix's Differential and Integral
Calculus.
According to the CRC Concise Encyclopedia of Mathematics (2003),
Euler used γ in 1781.
Gauss used ψ.
Julio González Cabillón has found γ in "Theoriae logarithmi
integralis lineamenta nova," an essay submitted by Carl Anton
Bretschneider (1808-1878) on October 13, 1835, to Crelle's
Journal. The article was published in volume 17, pp. 257-285,
1837. The symbol itself can be found on page 260.
DeMorgan used γ,
according to J. W. L. Glaisher in "On the History of Euler's
Constant" (1872) in The Messenger of Mathematics.
W. Shanks used "E. or Eul. constant" in Proc. Royl. Soc. of
London, Vol XV (1867).
The letter E was adopted by J. W. L. Glaisher in 1871
and J. C. Adams in 1878.
Ernst Pascal retained Mascheroni's notation A in 1900 (Cajori
vol. 2, page 32).
In vol. VII of "L'Intermediaire des mathematiciens" (1900), G. Vacca
(from Turin) asks who introduced γ. His question #1998 reads as follows:
In the German "Encyclopaedie" (1900, vol. II, p. 171) it
says that Mascheroni has denoted the Euler's constant 0.577... by
γ. According to my
research, this author designated it by letter "A".
In "Synopsis" of Mr. Hagen (1891, vol. I, p. 86), it is said that
Euler has introduced this symbol in "Acta Petr." (1769, vol. XIV).
Mr. E. Pascal, in his "Repertorium" (1900, vol. I, p. 478 of the
German edition) reproduces that suggestion. But, in the quoted volume,
and in many memoirs of Euler, I have found that this author has just
used the symbol "C", 'et parfois' "O".
Who is the first mathematician that has introduced the symbol γ for the Euler's constant?
In his Adnotationes ad calculum integrale Euleri (1790),
Lorenzo Mascheroni (1750-1800) calculated the constant to 32 decimal
places:
.57721 56649 01532 86061 81120 90082 39...
In 1809 Johann von Soldner (1766-1833) published his "Théorie
d'un nouvelle fonction transcendante," in which his value of the
constant given on page 13 is:
.57721 56649 01532 86060 6065...
which differs from Mascheroni's value at the twentieth decimal place.
In 1812 Gauss asked F. G. B. Nicolai (1793-1846), "juvenem in calculo
indefessum," to check their results, and he agreed with von Soldner.
A note in a memoir by Gauss which contained the results of Nicolai's
calculation apparently attracted little attention and Mascheroni's
value was repeatedly quoted thereafter (Glaisher).
φ for the golden ratio. According
to The Curves of Life: Being an Account of Spiral Formations and
Their Application to Growth in Nature, to Science, and to Art: With
Special Reference to the Manuscripts of Leonardo da Vinci (1914)
by Sir Theodore Andrea Cook (1867-1928), page 420:
Mr. Mark Barr . . . suggested . . . that this ratio
should be called the phi proportion for reasons given below . . .
The symbol phi was given to this proportion partly because it has a
familiar sound to those who wrestle constantly with pi and partly
because it is the 1st letter of the name of Pheidias, in whose
sculpture this sculpture is seen to prevail when the distance between
salient points are measured.
The above quotation and citation were provided by Samuel S. Kutler
and Julio González Cabillón. Barr was an American
mathematician.
According to Gardner (1961) and Huntley, the letter phi was chosen
because it is the first letter in the name of Phidias who is believed
to have used the golden proportion frequently in his sculpture.
However, Schwartzman (page 164) implies the letter stands for
Fibonacci.
The Greek letter tau is also used for this constant. Tau is found in
1948 in Regular Polytopes by Harold Scott MacDonald Coxeter,
according to John Conway, who believes Coxeter may have used the
symbol in his papers of the 1920s and 1930s. Ball and Coxeter (1987,
page 57) write, "The symbol [tau] is appropriate because it is the
initial of tomh\ ("section") [Antreas P. Hatzipolakis].
H. v. Baravalle used G for 0.618... in "The Geometry of the
Pentagon and the Golden Section," which appeared in The
Mathematics Teacher in January 1948. He may have used the same
symbol in his "Die Geometrie des Pentagrammes und der Goldene
Schnitt" in 1932.
In The Shape of the Great Pyramid (1999), Roger Herz-Fischler
uses G for 1.618... and g for .618....
i for the imaginary unit was first used by
Leonhard Euler (1707-1783) in a memoir presented in 1777 but not
published until 1794 in his "Institutionum calculi integralis."
On May 5, 1777, Euler addressed to the 'Academiae' the paper "De
Formulis Differentialibus Angularibus maxime irrationalibus quas tamen
per logarithmos et arcus circulares integrare licet," which was
published posthumously in his "Institutionum calculi integralis,"
second ed., vol. 4, pp. 183-194, Impensis Academiae Imperialis
Scientiarum, Petropoli, 1794.
Quoniam mihi quidem alia adhuc via non patet istud
praestandi nisi per imaginaria procedendo, formulam 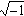 littera i in posterum
designabo, ita ut sit ii = -1 ideoque 1/i = -i.
littera i in posterum
designabo, ita ut sit ii = -1 ideoque 1/i = -i.
According to Cajori, the next appearance of i in print is by
Gauss in 1801 in the Disquisitiones Arithmeticae. Carl Boyer
believes that Gauss' adoption of i made it the standard. By
1821, when Cauchy published Cours d'Analyse, the use of
i was rather standard, and Cauchy defines i as "as if
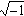 was a real quantity
whose square is equal to -1."
was a real quantity
whose square is equal to -1."
Throughout his Introductio, Euler consistently writes 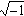 , denoting by i the "numerus
infinite magnus" [namely, an infinitely large number]. Nonetheless,
there are very few occasions where Euler chose i with a
different meaning. Thus, chapter XXI (volume 2) of Euler's
Introductio contains the first appearance of i as
quantitas imaginaria:
, denoting by i the "numerus
infinite magnus" [namely, an infinitely large number]. Nonetheless,
there are very few occasions where Euler chose i with a
different meaning. Thus, chapter XXI (volume 2) of Euler's
Introductio contains the first appearance of i as
quantitas imaginaria:
Cum enim numerorum negativorum Logarithmi sint imaginarii
(...) erit log(-n) quantitas imaginaria, quae sit = i.
The citation above is from "Introductio in analysin infinitorum,"
Lausannae, Apud Marcum-Michaelem Bousquet & socios, M.DCC.XLVIII
(1748).
Please note that, in this fascinating passage about logarithms, Euler
does not introduce the symbol i such that
i2 = -1.
[This entry was contributed by Julio González Cabillón.]
Older symbols for zero. The following is taken from a paper
"Africa, Cradle of Mathematics" by Beatrice Lumpkin:
It is well known that a zero placeholder was not used or
needed in Egyptian numerals, a system of numerals without place
value. Still historians such as Boyer and Gillings have found
examples of the use of the zero concept in ancient Egypt. But
Gillings added, "Of course zero, which had not yet been invented, was
not written down by the scribe or clerk; in the papyri, a blank space
indicates zero." However, some Egyptologists did know that the
ancient Egyptians used a zero symbol, but it may have been missed by
historians of mathematics because the symbol did not appear in the
surviving mathematical papyri.
The Egyptian zero symbol was a triliteral hieroglyph, with consonant
sounds [symbol]. This was the same hieroglyph used to represent
beauty, goodness, or completion. There are two major sources of
evidence for an Egyptian zero symbol:
1. Zero reference level for construction guidelines. Massive
stone structures such as the ancient Egyptian pyramids required deep
foundations and careful leveling of the courses of stone. Horizontal
leveling lines were used to guide the construction. One of these
lines, often at pavement level, was used as a reference and was
labeled [symbol], or zero. Other horizontal leveling lines were
spaced 1 cubit apart and labeled as 1 cubit above [symbol], 2 cubits
above [symbol], or 1 cubit, 2 cubits, 3 cubits, and so forth, below
[symbol]. Here zero was used as a reference for directed or signed
numbers.
In 1931, George Reisner described the terms used to label the
leveling lines at the Mycerinus (Menkure) pyramid at Giza, built c.
2600 BCE. He gave the following list collected earlier by Borchardt
and Petrie from their study of Old Kingdom pyramids. [...]
2. Bookkeeping, zero remainders. A bookkeeper's record from
the 13th dynasty c 1700 BCE shows a monthly balance sheet for items
received and disbursed by the royal court during its travels. On
subtracting total disbursements from total income, a zero remainder
was left in many columns. This zero remainder was represented with
the same symbol, [symbol], as used for the zero reference line in
construction.
These practical applications of a zero symbol in ancient Egypt, a
society which conventional wisdom believed did not have a zero, may
encourage historians to reexamine the everyday records of ancient
cultures for mathematical ideas that have been
overlooked.
According to Milo Gardner, Mesoamericans used a fully positional base
4, 5 system, with zero as a place holder, counting 0-19, as early as
1,000 BC.
As early as the fourth century BC, the Chinese represented zero as a
blank space on a counting board (Johnson, page 160).
Babylonians in the Seleucid period (300 BC onward) used a symbol for
zero, mainly in astronomical texts, and never in final positions.
According to Neugebauer in The Exact Sciences in Antiquity,
Dover, 2nd edition, 1969, p. 20:
The Babylonian place value notation shows in its earlier
development two disadvantages which are due to the lack of a symbol
for zero. The first difficulty consists in the possibility of
misreading a number 1 20 as 1,20 = 80 when actually 1,0,20 = 3620 was
meant. Occasionally this ambiguity is overcome by separating the two
numbers very clearly if a whole sexagesimal place is missing. But this
method is by no means strictly applied and we have many cases where
numbers are spaced widely without any significance. In the latest
period, however, when astronomical texts were computed, a special
symbol for "zero" was used. This symbol also occurs earlier as a
separation mark between sentences, and I therefore transcribe it by a
"period". Thus we find in Seleucid astronomical texts many instances
of numbers like 1, . , 20 or even 1, . , . ,20 which apply exactly the
same principle as, e.g.,our 201 or 2001.
But even in the final phase of Babylonian writing we do not find any
examples of zero signs at the end of numbers. Though there are many
instances of cases like . ,20 there is no safe example of a writing like
20, . known to me. In others words, in all periods the context alone
decides the absolute value of a sexagesimal written number.
However, Georges Iffrah (Histoire Universelle des Chiffres, Seghers,
Paris, 1981, p. 400-401) writes that Babylonian astronomers used the
zero not only in the intermediate positions but also in initial or
final positions, and he gives Neugebauer as the source of this
information.
According to Boyer (p. 29-30), "The Babylonians seem at first to have
had no clear way in which to indicate an "empty" position--that is,
they did not have a zero symbol, although they sometimes left a space
where a zero was intended. ... By about the time of the conquest by
Alexander the Great, however, a special sign, consisting of two small
wedges placed obliquely, was invented to serve as a placeholder where
a numeral was missing. ... The Babylonian zero symbol apparently did
not end all ambiguity, for the sign seems to have been used for
intermediate empty positions only. There are no extant tablets in
which the zero sign appears in a terminal position. This means that
the Babylonians in antiquity never achieved an absolute positional
system."
Burton says that about A. D. 150, the Alexandrian astronomer Ptolemy
began using the omicron [which looks something like a zero] in the
manner of our zero, not only in a medial but also in a terminal
position. He says there is no evidence that Ptolemy regarded the
symbol as a number by itself that could enter into computations with
other numbers. Omicron is the first letter of the Greek word for
"nothing." However, Len Berggren says, "Ptolemy probably did not use
omicron to denote 0. Papyri from the period when Ptolemy lived show a
small 'o' with a bar over it as the symbol for 0, and the small
'o' alone doesn't come in until the Byzantine period. Even in that
period Neugebauer considers it unlikely that the small 'o' stood for
the Greek word ouden (= nothing). See the discussion in the
second edition of Neugebauer's Exact Sciences in Antiquity, esp. pp.
13 - 14."
The oldest Maya artifact employing both positional notation and a zero
is Pestac, Stela 1, with a contemporaneous date of Feb. 8, AD 665. The
oldest Maya artifacts employing a zero but not positional notation are
Uaxactun, Stelae 18 and 19, with a contemporaneous date of AD 357. The
oldest Maya artifact employing the same chronological system as in the
previous cases but without a zero and without positional notation is
Tikal, Stela 29, with a contemporaneous date of July 8, AD 292
(Michael Closs).
[This website previously showed what seemed to be an occurrence of a
symbol for zero on a tablet from the Old Babylonian period, about
1800 B. C. However, it is now believed that this sole appearance was
not real and may have been due to an error of the scribe who made the
tablet.]
The Hindu zero symbol. The Encyclopaedia
Britannica says, "Hindu literature gives evidence that the zero
may have been known before the birth of Christ, but no inscription has
been found with such a symbol before the 9th century."
According to Johnson (page 160), by the third century A.D., Hindu
mathematicians were using a heavy dot to mark its place in
calculations and the dot was eventually replaced by an empty circle.
The earliest date Bell has found to be fairly proven for the use of
zero was A. D. 505 in a Pancasiddhantika by Varahamihira.
According to Iffrah (op. cit., p. 468), a heavy point for zero appears
in a Khmer inscription of 683 found at Trapeang Bay, Sambor Province,
Cambodia. A small circle for zero appears in 683 in an inscription
found in Kedukan Bukit, Palebang, Sumatra. A small circle is also
found in 684 at Talang Tuwo, Palebang, Sumatra, and in 686 at Kota
Kapur, Banka Island.
According to Menninger (p. 400):
This long journey begins with the Indian inscription which
contains the earliest true zero known thus far (Fig. 226). This famous
text, inscribed on the wall of a small temple in the vicinity of
Gvalior (near Lashkar in Central India) first gives the date 933 (A.D.
870 in our reckoning) in words and in Brahmi numerals. Then it goes on
to list four gifts to a temple, including a tract of land "270 royal
hastas long and 187 wide, for a flower-garden." Here, in the
number 270 the zero first appears as a small circle (fourth line in
the Figure); in the twentieth line of the inscription it appears once
more in the expression "50 wreaths of flowers" which the gardeners
promise to give in perpetuity to honor the divinity.
Manoel Almeida reports that, following Iffrah, the date of the Gvalior
inscription is A. D. 876. According to Johnson (page 160), the date
of the inscription is A. D. 840. Johnson says this inscription is the
oldest surviving use of an empty circle for zero.
Michael Closs writes that the Gvalior inscription is not the "earliest
known written zero" from India:
There are several more ancient inscriptions which also
exhibit written zeros within the context of positional notation.
Rabindra Nath Mukherjee, an Indian historian of mathematics, has noted
several such artifacts. Unfortunately, he has provided neither
photographs of the artifacts nor descriptions of the texts. I am
hoping to collect this information. The oldest hard evidence which
Mukherjee gives is an inscription from AD 672 in which the zero is
written as a small dot. Next is an inscription from AD 683 in which
the zero is written as a large dot. This is followed by an inscription
from AD 684 in which the zero is written as a small circle. This is
the earliest known (by me!) antecedent having the same form as our
modern zero symbol.
Iffrah (op. cit., p. 464-465) mentions two cooper's letters, dated from
VIII AD, with small circles to represent zeros. Both are earlier than
Gwalior inscriptions, but consensus about its authenticity is
lacking.
Manoel Almeida, who
contributed much of the information for this article, says he
suspects, due to the puzzling coincidence of dates and of the use of
the same symbols for zero, that the AD 683 and AD 684 inscriptions
mentioned by Mukherjee can be the Trapeang Bay and the Talang Tuwo
inscriptions, quoted by Iffrah. He is interested in additional
information from Mukherjee's articles.
Another source says the first zero in the Hindu system was represented
by a dot and was found in a text written by Bakhshali, the date of
which is unknown. The Bakhshali manuscript may have been written in
the 8th or 9th century, but may have been written later and may not
even be of Hindu origin (Smith, vol. 1, page 164).
According to Georges Iffrah in Histoire Universelle des Chiffres,
Seghers, Paris, 1981, p.489-490, the first European to advocate the
use of the Hindu zero was Abraham ben Meir ibn Ezra (1092-1167), who
wrote Sfer H Mispar (The Book of the Number), in which he used
the circle to represent zero. He preferred to use the first nine
letters of the Hebrew alphabet rather than the nine Hindu-Arabic
numbers. He called the zero Galgal (Hebrew for wheel) or Sifra
(apud the arab Sifr, certainly). He also changed the old Hebrew
alphabetic numeration system into a new decimal positional system like
ours.
Leonardo of Pisa (1180-1250) (or Fibonacci) also advocated the use of
zero, using the term zephirum in Liber Abaci.
The zero symbol first appears in print in the 1200s, according to
Burton. Smith says that Ch'in Kiu-shao (or Tsin Kiu tschaou or Ts'in
K'ieou-Chao) of China used 0 in 1247 or 1257 in The Nine
Sections of Mathematics.
Rida A. K. Irani, in a paper in Centaurus, vol. 4 (1955), pp. 1-12
gives forms of the Hindu-Arabic characters as they occur in dated
Arabic manuscripts, and shows that the medieval Arabs used a small 'o'
for zero. This tends to degenerate to a dot in late (e.g. 17th
century) manuscripts.
Front Page |
Operation |
Grouping |
Relation |
Fractions and Decimals |
Constants |
Variables |
Functions |
Geometry |
Trigonometry |
Calculus |
Matrices and Vectors |
Set Theory and Logic |
Number theory |
Statistics |
Sources
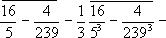 , &c. = 3.14159, &c. = π. This series (among others for the
same purpose, and drawn from the same Principle) I received from the
Excellent Analyst, and my much esteem'd Friend Mr. John Machin;
and by means thereof, Van Ceulen's Number, or that in Art.
64.38 may be Examin'd with all desirable Ease and Dispatch."
, &c. = 3.14159, &c. = π. This series (among others for the
same purpose, and drawn from the same Principle) I received from the
Excellent Analyst, and my much esteem'd Friend Mr. John Machin;
and by means thereof, Van Ceulen's Number, or that in Art.
64.38 may be Examin'd with all desirable Ease and Dispatch."


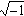 littera i in posterum
designabo, ita ut sit ii = -1 ideoque 1/i = -i.
littera i in posterum
designabo, ita ut sit ii = -1 ideoque 1/i = -i.